qsl_courses-removebg-preview
3.1. AC – peak, peak-to-peak, average, RMS

Understanding AC Measurements

This chapter introduces the foundational concepts of alternating current (AC) measurements, vital for anyone involved in electrical engineering or working with electronic devices. The focus is on understanding various amplitude dimensions of AC signals, such as peak, peak-to-peak, average, and RMS (Root Mean Square) values. These measurements are crucial for accurately analyzing and interpreting AC waveforms, especially when viewed through devices like oscilloscopes. The chapter also delves into how these measurements are used in practical applications, including the calibration of AC voltmeters and the application of Ohm’s law in AC circuits. By grasping these concepts, learners gain a comprehensive understanding of how AC voltages and currents are measured and interpreted, laying a foundation for more advanced studies in electronics and electrical systems.

  • A-003-001-001: What is the easiest amplitude dimension to measure by viewing a pure sine wave on an oscilloscope?

    Understanding AC Amplitude Measurements (A-003-001-001)

    Measuring Amplitude of a Sine Wave on an Oscilloscope:
    Question A-003-001-001 addresses the easiest amplitude dimension to measure when viewing a pure sine wave on an oscilloscope, with the correct answer being A) Peak-to-peak voltage. The peak-to-peak voltage is the simplest amplitude measurement on an oscilloscope for a sine wave, as it represents the full height of the wave from its highest to its lowest point. This measurement is straightforward because it requires visually identifying and measuring the vertical distance between the two extreme points of the wave on the oscilloscope’s display.

    Parallels:

    1. Measuring the Height of a Wave: Similar to measuring the total height of a sea wave from trough to crest.
    2. Ruler Measurement: Like using a ruler to measure the total height of an object from its bottom to top point.

    Question Summary and Key Takeaways:

    1. Peak-to-Peak Measurement: Easiest to measure the peak-to-peak voltage of a sine wave on an oscilloscope.
    2. Visual Identification: Involves visually identifying the highest and lowest points.
    3. Total Wave Height: Represents the total vertical distance of the wave.
    4. Simple and Direct: A straightforward method for amplitude measurement.
    5. Useful in Signal Analysis: Essential for analyzing signal amplitude in various applications.

    A-003-001-001: What is the easiest amplitude dimension to measure by viewing a pure sine wave on an oscilloscope?

    Your score is

  • A-003-001-002: What is the RMS value of a 340 volt peak-to-peak pure sine wave?

    RMS Value of a Sine Wave (A-003-001-002)

    Calculating RMS Voltage of a Sine Wave:
    Question A-003-001-002 asks about the RMS value of a 340 volt peak-to-peak pure sine wave, with the correct answer being C) 120 volts. The RMS (Root Mean Square) value represents the effective power of an AC voltage and, for a pure sine wave, is approximately 0.707 times the peak voltage. For a 340 volt peak-to-peak sine wave, which is double the peak voltage, the peak voltage is 170 volts (340 volts divided by 2), leading to an RMS voltage of about 120 volts (170 volts times 0.707). See below….

    Parallels:

    1. Average Speed of a Car: Think of RMS voltage like calculating the average speed of a car over a journey, representing an effective measure of speed (voltage).
    2. Heat Produced by a Light Bulb: Similar to the consistent heat produced by a light bulb, regardless of fluctuations in power, RMS voltage represents the consistent power delivery of an AC source.

    Question Summary and Key Takeaways:

    1. RMS as Effective Voltage: RMS value represents the effective power of an AC voltage.
    2. Calculation from Peak-to-Peak: Derived from peak voltage, which is half of the peak-to-peak voltage.
    3. 0.707 Times the Peak Voltage: RMS for a sine wave is about 0.707 times its peak voltage.
    4. Useful in Power Calculations: RMS value is crucial for calculating the power delivered by AC sources.
    5. Standard in Electrical Engineering: A standard measure used in electrical engineering for AC circuits.

    A-003-001-002: What is the RMS value of a 340 volt peak-to-peak pure sine wave?

    Your score is

  • A-003-001-003: What is the equivalent to the RMS value of an AC voltage?

    Equivalent of RMS Value in AC Voltage (A-003-001-003)

    Understanding RMS Value in AC Circuits:
    Question A-003-001-003 explores what the RMS value of an AC voltage is equivalent to, with the correct answer being D) The AC voltage causing the same heating of a given resistor as a DC voltage of the same value. The RMS (Root Mean Square) value of an AC voltage is the equivalent DC voltage that would produce the same amount of heat in a resistor. This comparison of the ‘effectiveness’ of AC and DC voltages in terms of power delivery is practical and essential in various electrical applications. The RMS value allows for direct comparisons between AC and DC, particularly in terms of their ability to deliver power to a load, like a resistor.

    Parallels:

    1. Consistent Heating with Different Stoves: Like different stoves (AC and DC sources) producing the same level of heat in a pot, RMS value represents the equivalent heating effect.
    2. Equal Intensity of Different Lights: Similar to different types of light bulbs (AC and DC powered) providing the same level of brightness.

    Question Summary and Key Takeaways:

    1. RMS as Equivalent DC Value: RMS value is equivalent to a DC voltage in terms of heating effect.
    2. Comparison of AC and DC: Allows for direct comparison between the effectiveness of AC and DC voltages.
    3. Practical in Electrical Applications: Useful for practical comparisons and calculations involving AC and DC.
    4. Reflects Power Delivery: Indicates the power delivery capability of an AC voltage.
    5. Crucial for Electrical Engineering: An important concept in electrical engineering and circuit design.

     

    A-003-001-003: What is the equivalent to the RMS value of an AC voltage?

    Your score is

  • A-003-001-004: If the peak value of a 100 Hz sinusoidal waveform is 20 volts, the RMS value is:

    RMS Value of a 100 Hz Sinusoidal Waveform (A-003-001-004)

    Calculating the RMS Value of a Sinusoidal Waveform:
    Question A-003-001-004 focuses on determining the RMS value of a 100 Hz sinusoidal waveform with a peak value of 20 volts, with the correct answer being A) 14.14 volts. The RMS (Root Mean Square) value for a sinusoidal waveform is approximately 0.707 times its peak value. Therefore, for a waveform with a peak voltage of 20 volts, the RMS value is calculated as 20 volts multiplied by 0.707, resulting in approximately 14.14 volts. This RMS value signifies the waveform’s effective voltage, similar to a DC voltage in terms of the power it can deliver to a load, such as a resistor.

    Parallels:

    1. Average Depth of a Pool: Imagine measuring the average depth of a wavy pool; the RMS value is like finding the consistent depth that represents the overall effect.
    2. Consistent Temperature in Cooking: Similar to maintaining a consistent temperature in cooking, the RMS value represents the effective ‘heat’ or power of the AC waveform.

    Question Summary and Key Takeaways:

    1. RMS Value Calculation: The RMS value of a sinusoidal waveform is about 0.707 times the peak value.
    2. Effective Voltage Representation: RMS value represents the effective voltage of the waveform.
    3. Equivalent to DC Power Delivery: The RMS value equates to a DC voltage in terms of power delivery capabilities.
    4. Useful in Electrical Applications: Important for calculations involving AC power and circuit design.
    5. Standard Measurement in AC Circuits: A standard and essential measure in AC circuit analysis.

    A-003-001-004: If the peak value of a 100 Hz sinusoidal waveform is 20 volts, the RMS value is:

    Your score is

  • A-003-001-005: In applying Ohm’s law to AC circuits, current and voltage values are:

    Applying Ohm’s Law to AC Circuits (A-003-001-005)

    Use of RMS Values in AC Ohm’s Law Calculations:
    Question A-003-001-005 asks about the values used in applying Ohm’s law to AC circuits, with the correct answer being D) peak values times 0.707. In AC circuits, Ohm’s law (V = IR) is commonly applied using RMS values for current (I) and voltage (V). The RMS value of a sinusoidal AC waveform is approximately 0.707 times its peak value. This factor is utilized to convert peak values to RMS values, enabling accurate application of Ohm’s law in AC circuits, ensuring that the voltage and current values used reflect the actual power delivery and resistance interactions in the circuit.

    Parallels:

    1. Average Speed for a Journey: Like using an average speed to calculate travel time, RMS values provide a consistent measure for applying Ohm’s law in AC circuits.
    2. Consistent Temperature for Baking: Comparable to using a consistent oven temperature for baking, RMS values offer a standardized approach for electrical calculations.

    Question Summary and Key Takeaways:

    1. RMS Values in AC Ohm’s Law: RMS values are used for voltage and current in AC Ohm’s law calculations.
    2. Conversion from Peak Values: RMS values are about 0.707 times the peak values.
    3. Reflects Actual Power Delivery: This approach accurately reflects the power delivery in AC circuits.
    4. Standardized for AC Analysis: RMS values offer a standardized method for electrical analysis in AC.
    5. Crucial for Circuit Calculations: Essential for accurate electrical calculations in AC circuits.

    A-003-001-005: In applying Ohm's law to AC circuits, current and voltage values are:

    Your score is

  • A-003-001-006: The effective value of a sine wave of voltage or current is:

    Effective Value of a Sine Wave (A-003-001-006)

    RMS as the Effective Value of a Sine Wave:
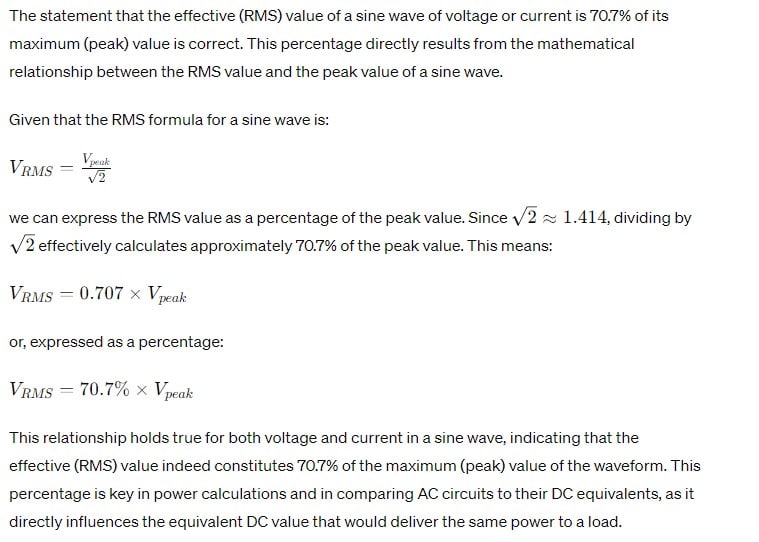
    Question A-003-001-006 addresses the effective value of a sine wave of voltage or current, with the correct answer being C) 70.7% of the maximum value. The effective value, also known as the RMS (Root Mean Square) value, of a sine wave is approximately 70.7% of its maximum (peak) value. This percentage represents the equivalent DC value in terms of the power that the AC wave can deliver, making it a crucial concept in AC power systems. The RMS value allows for meaningful comparisons and calculations involving AC and DC, particularly in terms of their capability to deliver power to a load.

    Parallels:

    1. Steady Jogging Pace: The RMS value is like maintaining a steady jogging pace that represents an average level of effort, equivalent to 70.7% of a maximum sprint.
    2. Constant Brightness of a Lamp: Similar to a lamp that provides a consistent level of brightness, representing an effective illumination level.

    Question Summary and Key Takeaways:

    1. RMS as Effective Value: RMS value is the effective value of a sine wave, about 70.7% of its peak.
    2. Equivalent to DC Power: Represents the equivalent power delivery as a DC voltage.
    3. Standard in Power Calculations: A standard measure used in power calculations for AC circuits.
    4. Useful for Comparing AC and DC: Allows for meaningful comparisons between AC and DC.
    5. Essential in Electrical Engineering: A fundamental concept in electrical engineering and circuit design.

    A-003-001-006: The effective value of a sine wave of voltage or current is:

    Your score is

  • A-003-001-007: AC voltmeter scales are usually calibrated to read:

    AC Voltmeter Scale Calibration (A-003-001-007)

    Understanding AC Voltmeter Readings:
    Question A-003-001-007 inquires about the calibration scale of AC voltmeters, with the correct answer being C) RMS voltage. AC voltmeters are typically calibrated to read RMS (Root Mean Square) voltage. This calibration is crucial because the RMS value of an AC voltage is a practical measure that represents the effective power of the voltage, similar to a DC voltage. By calibrating voltmeters to read RMS values, it ensures a standardized and meaningful measurement of AC voltage in electrical applications, providing a reliable indication of the voltage’s actual impact on a load, such as a resistor.

    Parallels:

    1. Thermometer Reading for Average Temperature: Think of an AC voltmeter reading RMS voltage like a thermometer showing the average room temperature, giving a practical sense of warmth.
    2. Speedometer Showing Average Speed: Similar to a speedometer showing the average speed of a car, the RMS reading on an AC voltmeter provides a practical and effective measure of voltage.

    Question Summary and Key Takeaways:

    1. RMS Voltage Calibration: AC voltmeters are calibrated to read RMS voltage.
    2. Effective Power Measurement: RMS value represents the effective power of an AC voltage.
    3. Comparable to DC Voltage: RMS voltage is a useful measure, similar to a DC voltage in terms of heating effect.
    4. Standardization in Measurements: Ensures standardized and meaningful voltage measurements.
    5. Essential in Electrical Engineering: RMS calibration is crucial for accurate electrical measurements and analyses.

    A-003-001-007: AC voltmeter scales are usually calibrated to read:

    Your score is

  • A-003-001-008: An AC voltmeter is calibrated to read the:

    Calibration of an AC Voltmeter (A-003-001-008)

    Effective Value Measurement by AC Voltmeters:
    Question A-003-001-008 asks about the calibration of an AC voltmeter, with the correct answer being D) effective value. An AC voltmeter is calibrated to read the effective value of an AC voltage, which corresponds to the RMS (Root Mean Square) value. This effective value is significant because it reflects the actual power or heating effect of the AC voltage, comparable to a DC voltage of the same magnitude. This calibration makes the voltmeter readings more meaningful and practical for electrical applications, as it provides an accurate representation of the voltage’s impact on electrical components.

    Parallels:

    1. Weighing Scale for Average Weight: Like a weighing scale showing the average weight, an AC voltmeter calibrated for effective value provides a realistic measure of voltage impact.
    2. Average Daily Temperature Reading: Similar to how meteorologists report the average daily temperature, an AC voltmeter’s effective value reading gives a practical sense of the voltage’s impact.

    Question Summary and Key Takeaways:

    1. Calibrated for Effective Value: AC voltmeters are calibrated to read the effective (RMS) value.
    2. Realistic Power Measurement: The effective value represents the real power impact of the AC voltage.
    3. Comparable to DC Measurements: Offers a practical comparison with equivalent DC voltage.
    4. Useful in Practical Applications: Essential for accurate and meaningful voltage readings in electrical systems.
    5. Standard in Electrical Measurements: This calibration is a standard in electrical engineering for precise and reliable voltage assessments.

    A-003-001-008: An AC voltmeter is calibrated to read the:

    Your score is

  • A-003-001-009: Which AC voltage value will produce the same amount of heat as a DC voltage, when applied to the same resistance?

    Comparison of AC and DC Heating Effects (A-003-001-009)

    RMS Value in Heat Production:
    Question A-003-001-009 explores which AC voltage value produces the same amount of heat as a DC voltage when applied to the same resistance, with the correct answer being C) The RMS value. The RMS (Root Mean Square) value of an AC voltage is the equivalent to a DC voltage in terms of the heating effect it produces when applied to a resistor. In practical terms, an AC voltage with a specific RMS value will generate the same amount of heat in a resistor as a DC voltage of the same value. This equivalence makes the RMS value a crucial parameter for comparing and analyzing the effects of AC and DC voltages in electrical applications, particularly in power delivery and heating.

    Parallels:

    1. Consistent Cooking Heat: Like two different stoves (AC and DC sources) providing the same level of heat for cooking, RMS voltage indicates equivalent heating effects.
    2. Uniform Lighting Intensity: Similar to different types of lamps providing the same brightness level, regardless of their power source.

    Question Summary and Key Takeaways:

    1. RMS Equivalent to DC in Heating: RMS value of AC is equivalent to DC voltage in terms of heating effect.
    2. Practical Comparison Between AC and DC: Allows for direct comparison of AC and DC in power applications.
    3. Key in Power Analysis: Crucial for analyzing and designing power delivery systems.
    4. Standard Parameter in Electrical Engineering: Widely used and accepted measure in electrical applications.
    5. Essential for Circuit Design: Important in designing circuits and choosing components for specific power requirements.

    A-003-001-009: Which AC voltage value will produce the same amount of heat as a DC voltage, when applied to the same resistance?

    Your score is

  • A-003-001-010: What is the peak-to-peak voltage of a sine wave that has an RMS voltage of 120 volts?

    Peak-to-Peak Voltage from RMS Value (A-003-001-010)

    Determining Peak-to-Peak Voltage:
    Question A-003-001-010 asks about finding the peak-to-peak voltage from an RMS voltage value, with the correct answer being C) 339.5 volts. To calculate the peak-to-peak voltage from an RMS voltage of a sine wave, one must first find the peak voltage by dividing the RMS value by approximately 0.707 (since RMS voltage is about 0.707 times the peak voltage). For an RMS voltage of 120 volts, the peak voltage would be 120 volts divided by 0.707, resulting in approximately 169.7 volts. The peak-to-peak voltage, which is double the peak voltage, would therefore be about 339.4 volts (169.7 volts times 2). This calculation is useful in various electrical and electronic applications for understanding the full range of voltage variation in AC circuits.

    Parallels:

    1. Total Elevation Change in Hiking: Like calculating the total elevation change in a hike from the base to the peak and back, the peak-to-peak voltage represents the total voltage swing.
    2. Full Swing of a Pendulum: Similar to measuring the full swing of a pendulum from one extreme to the other, representing the complete range of movement.

    Question Summary and Key Takeaways:

    1. Calculating from RMS Value: Peak-to-peak voltage is derived from the RMS value using a conversion factor.
    2. Double the Peak Voltage: Peak-to-peak voltage is twice the peak voltage.
    3. Reflects Total Voltage Range: Indicates the full range of voltage variation in the waveform.
    4. Useful in Circuit Analysis: Important for understanding voltage limits in AC circuits.
    5. Standard Calculation in AC Applications: A common calculation in electrical engineering and electronics.

    A-003-001-010: What is the peak-to-peak voltage of a sine wave that has an RMS voltage of 120 volts?

    Your score is

  • A-003-001-011: A sine wave of 17 volts peak is equivalent to how many volts RMS?

    RMS Value of a Sine Wave (A-003-001-011)

    Calculating RMS Voltage of a Sine Wave:
    Question A-003-001-011 pertains to finding the RMS voltage of a sine wave with a peak of 17 volts, with the correct answer being C) 12 volts. The RMS (Root Mean Square) value of a sine wave is approximately 0.707 times its peak voltage. Therefore, for a sine wave with a peak voltage of 17 volts, the RMS voltage is calculated as 17 volts multiplied by 0.707, resulting in approximately 12 volts. This RMS value represents the effective voltage of the AC waveform, akin to a DC voltage in terms of its power delivery capability to a load, such as a resistor.

    Parallels:

    1. Average Depth of a River: Imagine measuring the average depth of a river with varying depths; the RMS value is akin to finding the consistent depth that represents the overall water flow.
    2. Consistent Heat in Cooking: Similar to maintaining a consistent cooking temperature, the RMS value represents the effective ‘heat’ or power of the AC waveform.

    Question Summary and Key Takeaways:

    1. RMS Value Calculation: The RMS value of a sine wave is about 0.707 times its peak voltage.
    2. Effective Voltage Representation: RMS value represents the effective voltage of the waveform.
    3. Equivalent to DC Power Delivery: The RMS value equates to a DC voltage in terms of power delivery.
    4. Useful in Electrical Applications: Important for calculations involving AC power and circuit design.
    5. Standard Measurement in AC Circuits: A standard and essential measure in AC circuit analysis

    A-003-001-011: A sine wave of 17 volts peak is equivalent to how many volts RMS?

    Your score is

Exploring AC Measurements

In this chapter, the intricacies of measuring and interpreting AC signals were explored, focusing on various amplitude dimensions such as peak, peak-to-peak, average, and RMS values.

  • The easiest amplitude dimension to measure on an oscilloscope was identified as the peak-to-peak voltage, being the most visually straightforward on a sine wave.
  • Understanding the RMS value was a key theme, with explanations on how it represents the effective power of AC voltage. RMS values were shown to be crucial in various applications, such as calibrating AC voltmeters and applying Ohm’s law in AC circuits.
  • The chapter highlighted the conversion processes between different types of measurements, like determining the RMS value from peak-to-peak voltage and vice versa, enhancing the learner’s ability to navigate between different measurement standards.

By covering these foundational aspects of AC measurements, the chapter equipped learners with essential knowledge for accurately assessing and working with AC electrical systems, an important skill in both academic and practical electrical engineering contexts.

3.1 AC – peak, peak-to-peak, average, RMS

Welcome to the Chapter Quiz!

Remember, each question is an opportunity to apply the QSL method and solidify your understanding of each topic. Take your time, think it through, and enjoy the challenge.

You need a score of 70% to pass the Quiz, but why not take a bit more time to review the course content and ‘shoot’ for 100%. Simply review the material again and re-take this Quiz.

Best of luck!

73 Don VE7DXE

 

1 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-001: What is the easiest amplitude dimension to measure by viewing a pure sine wave on an oscilloscope?

2 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-002: What is the RMS value of a 340 volt peak-to-peak pure sine wave?

3 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-003: What is the equivalent to the RMS value of an AC voltage?

4 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-004: If the peak value of a 100 Hz sinusoidal waveform is 20 volts, the RMS value is:

5 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-005: In applying Ohm’s law to AC circuits, current and voltage values are:

6 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-006: The effective value of a sine wave of voltage or current is:

7 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-007: AC voltmeter scales are usually calibrated to read:

8 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-008: An AC voltmeter is calibrated to read the:

9 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-009: Which AC voltage value will produce the same amount of heat as a DC voltage, when applied to the same resistance?

10 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-010: What is the peak-to-peak voltage of a sine wave that has an RMS voltage of 120 volts?

11 / 11

Category: AC – peak, peak-to-peak, average, RMS

A-003-001-011: A sine wave of 17 volts peak is equivalent to how many volts RMS?

Your score is

0%